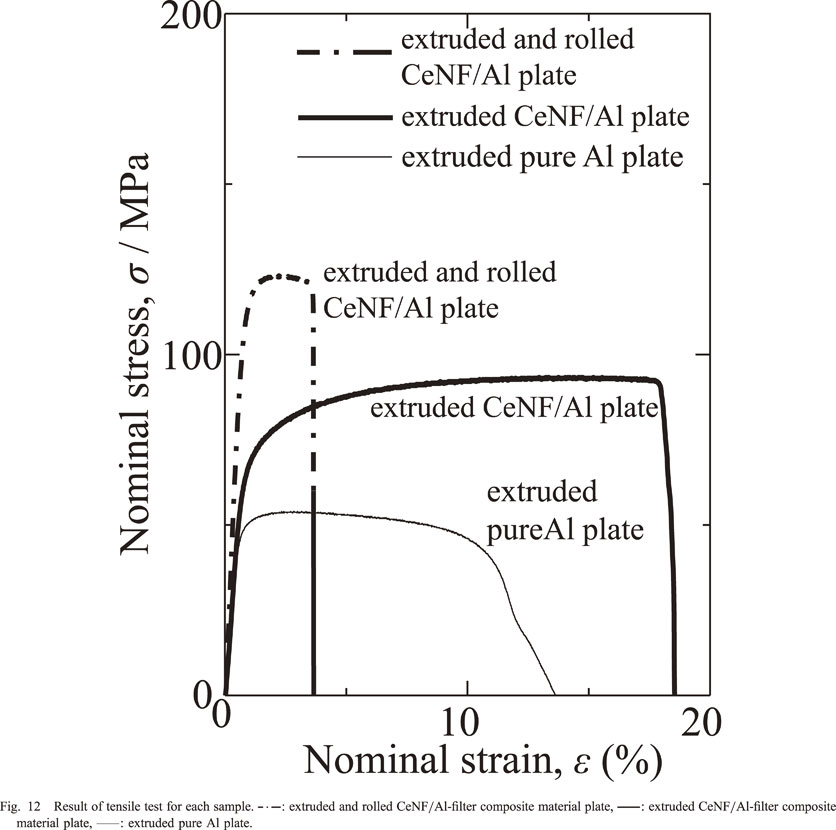
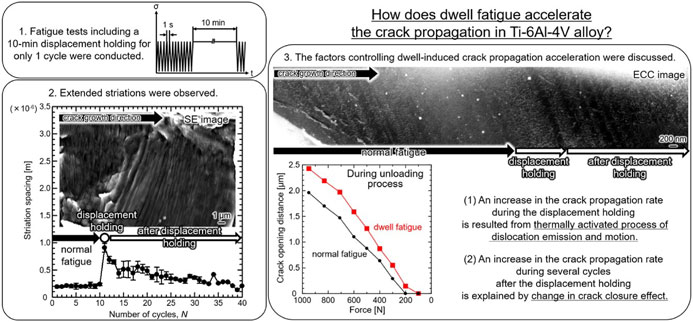
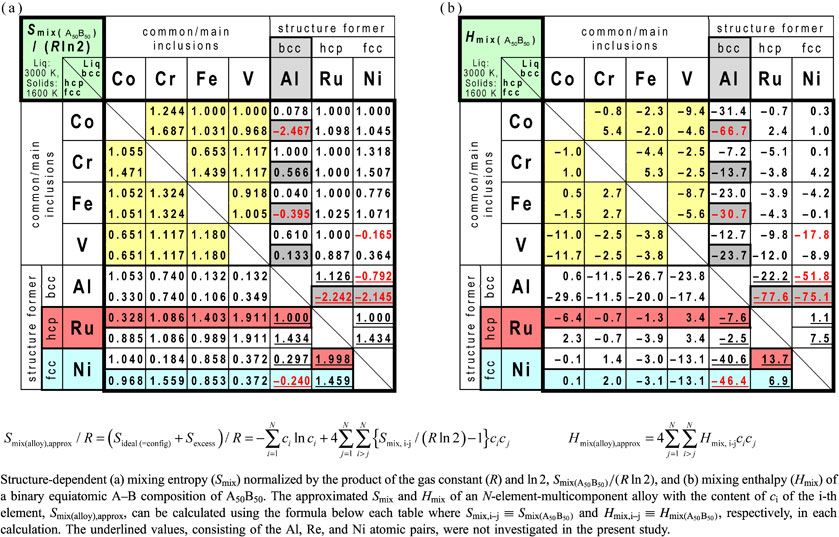
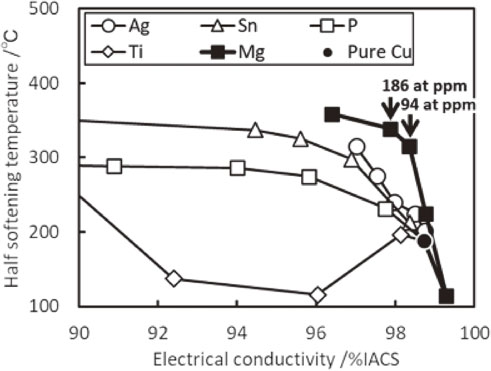
MATERIALS TRANSACTIONS
Materials Transactionsは、1960年にTransactions of The Japan Institute of
Metalsとして創刊し、2000年に材料系学協会との共同刊行を開始してMaterials
Transactionsと誌名を改め、現在は材料系14学協会が参加している英文学術論文誌です。金属及び関連する電子材料、磁気材料、超電導材料、セラミックス等の先端材料に関する研究成果を対象とする学術論文誌として、一般投稿論文、特集論文、Review、Overview等の記事を掲載し、金属や関連する材料に関する研究を国内外へ情報発信しています。
もっと読む
公益社団法人 日本金属学会
が発行
収録数 13,747本
(更新日 2024/04/19)
(更新日 2024/04/19)
Online ISSN : 1347-5320
Print ISSN : 1345-9678
ISSN-L : 1345-9678
Print ISSN : 1345-9678
ISSN-L : 1345-9678
1.200
2022 Journal Impact Factor (JIF)
2022 Journal Impact Factor (JIF)
ジャーナル
査読
認証あり
HTML
早期公開
Scopus
Scopus